Calculus III
Contents
3 Dimensional space
Partial derivatives
Multiple integrals
Vector Functions
Line integrals
Surface integrals
Vector operators
Applications
© The scientific sentence. 2010
|
|
Calculus III:
Line integrals of vector fields
1. Definition and method
Let's consider the vector field:
 (x,y,z) =
P(x,y,z) (x,y,z) =
P(x,y,z) + Q(x,y,z) + Q(x,y,z) + R(x,y,z) + R(x,y,z) ,
,
and the three-dimensional, smooth curve given by
 (t) =
x(t) (t) =
x(t) + y(t) + y(t) + z(t) + z(t)
With
a ≤ t ≤ b
The line integral of the vector  along the curve C is given by:
along the curve C is given by:
∫C . . 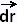 =
∫ab =
∫ab
 ( ( (t)
) . (t)
) . 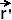 (t) dt (t) dt
This formula involves the the dot product of the vector field and the differential vector and the differential vector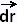
Note that
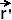 (t) dt = ( (t) dt = (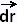 /dt) dt,
and /dt) dt,
and
 ( ( (t) ) = (t) ) =
 ( x(t),y(t),z(t) ) ( x(t),y(t),z(t) )
As a line integral with respect to arc length ds, the line integrals of vector fields takes the form:
∫C . . 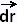 =
∫C =
∫C . .  ds ds
Where
 is the unit tangent vector given by is the unit tangent vector given by
 = = 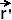 (t)/∥ (t)/∥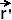 (t) ∥ (t) ∥
Example 1
Evaluate ∫C . . 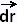
where  (x,y,z) = xy (x,y,z) = xy + xz + xz + yz + yz
and C is the curve given by :
 (t) = t (t) = t + (1/2)t2 + (1/2)t2 + (1/3)t3 + (1/3)t3
With 0 ≤ t ≤ 1
• 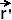 (t) = (t) = 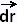 (t)/dt = (t)/dt =
 + t + t + t2 + t2 = =
〈 1, t, t2 〉
•  ( ( (t) ) = (t) ) =
 (t, (1/2)t2, (1/3)t3) = (t, (1/2)t2, (1/3)t3) =
〈 (1/2)t3, (1/3)t4, (1/6)t5 〉
Therefore:
∫C . . 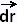 =
∫C =
∫C . . 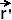 dt = dt =
∫01
〈(1/2)t3, (1/3)t4, (1/6)t5〉 .
〈 1, t, t2〉
dt =
∫01
((1/2)t3 + (1/3)t5 + (1/6)t7)
dt =
((1/4)t4 + (1/18)t6 + (1/48)t8)|01 =
1/4 + 1/18 + 1/48 =
13/48 + 1/18 =
47/144.
∫C . . 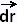 = 47/144 = 47/144
Example 2
Evaluate ∫C . . 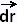 where where  (x,y,z) = xy (x,y,z) = xy  + xz + xz and C is the line segment from (-1 , 0, 1) and (- 2, 1, 2).
and C is the line segment from (-1 , 0, 1) and (- 2, 1, 2).
The parameterization for the line is
x(t) = -1(1 - t) - 2t = - 1 - t
y(t) = 0(1 - t) + 1 t = t
z(t) = 1(1 - t) + 2t = 1 + t
0 ≤ t ≤ 1
 ( ( (t) ) =
〈 - t(1 + t), 0, - (1 + t)2〉 (t) ) =
〈 - t(1 + t), 0, - (1 + t)2〉
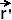 (t) =
〈 - 1, 1, 1 〉 (t) =
〈 - 1, 1, 1 〉
 ( ( (t) ) . (t) ) .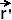 (t) = t(1 + t) + 0 - (1 + t)2 =
- 1 - t (t) = t(1 + t) + 0 - (1 + t)2 =
- 1 - t
Therefore
∫C . . 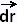 =
∫01( - 1 - t) dt = =
∫01( - 1 - t) dt =
( - t - t2/2)|01 =
( - 1 - 1/2) =
- 3/2.
∫C . . 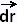 = - 3/2
= - 3/2
2. Line integrals of vector fields and
line integrals with respect to x, y, and z
Given the vector field
 (x,y,z) =
P(x,y,z) (x,y,z) =
P(x,y,z) + Q(x,y,z) + Q(x,y,z) + R(x,y,z) + R(x,y,z)
and the curve C parameterized by
 (t) =
( x(t),y(t),z(t) ), with a ≤ t ≤ b, (t) =
( x(t),y(t),z(t) ), with a ≤ t ≤ b,
the line integral is:
∫C . . 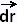 =
∫CP(x,y,z) dx + Q(x,y,z) dy + R(x,y,z) dz
=
∫CP(x,y,z) dx + Q(x,y,z) dy + R(x,y,z) dz
Which is another method for evaluating line integrals of vector fields.
Since a line integrals of vector fields can be defined in terms of line integrals with respect to the coordinates x, y, and z, then reversing the direction of the path gives the opposite
of of the line integral. That is:
∫- C  . . 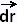 = - ∫C
= - ∫C  . . 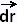
|
|