Calculus III
Contents
3 Dimensional space
Partial derivatives
Multiple integrals
Vector Functions
Line integrals
Surface integrals
Vector operators
Applications
© The scientific sentence. 2010
|
|
Calculus III:
Fundamental theorem for line integrals
1. Definition and method
In Calculus I we had used the fundamental theorem of calculus
that show us how to evaluate definite integrals. That is :
∫ab f'(x) dx = f(b) - f(a)
There is a version of this theorem for line integrals
over certain kinds of vector fields:
Theorem
Suppose that C is a smooth curve given by  (t),
a ≤ t ≤ b. Also suppose that f is a function of multiple
variable whose gradient vector ∇f is continuous on C. Then: (t),
a ≤ t ≤ b. Also suppose that f is a function of multiple
variable whose gradient vector ∇f is continuous on C. Then:
∫C∇f . 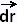 = f( = f( (b)) - f( (b)) - f( (a)) (a))
Note that  (a) represents the initial
point on C while (a) represents the initial
point on C while  (b) represents the
final point on C. (b) represents the
final point on C.
Indeed,
∫C∇f . 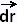 =
∫ab (∂f/∂x)dx + (∂f/∂y)dy + (∂f/∂z)dz = =
∫ab (∂f/∂x)dx + (∂f/∂y)dy + (∂f/∂z)dz =
∫ab (∂f/∂x)(dx/dt)dt + (∂f/∂y)(dy/dt)dt + (∂f/∂z)(dz/dt)dt =
∫ab [(∂f/∂x)(dx/dt) + (∂f/∂y)(dy/dt) + (∂f/∂z)(dz/dt)] dt =
∫ab (d[f( (t))]/dt) dt
=
∫ab df( (t))]/dt) dt
=
∫ab df( (t)) (t))
Using the Fundamental theorem of calculus for single integrals, we obtain:
∫C∇f . 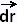 = f( = f(
 (b)) - f( (b)) - f( (a)) (a))
Example 1
Evaluate ∫C∇f . 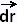 where f(x, y, z) = xy + xz + yz,
and C is any path from (- 1 , 0, 1) and (- 2, 1, 2). where f(x, y, z) = xy + xz + yz,
and C is any path from (- 1 , 0, 1) and (- 2, 1, 2).
We have then:
 (a) = 〈 - 1 , 0, 1 〉
, and (a) = 〈 - 1 , 0, 1 〉
, and
 (b) = 〈 - 2, 1, 2 〉 (b) = 〈 - 2, 1, 2 〉
f( (a)) = (- 1)(0) + (- 1)(1) + (0)(1) = - 1 (a)) = (- 1)(0) + (- 1)(1) + (0)(1) = - 1
f( (b)) = (- 2 )(1) + (- 2)(2) + (1)(2) = - 4 (b)) = (- 2 )(1) + (- 2)(2) + (1)(2) = - 4
Therefore
f( (b)) - f( (b)) - f( (a)) =
- 4 - (- 1) = - 3 (a)) =
- 4 - (- 1) = - 3
∫C ∇f . 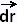 = - 3
= - 3
|
|